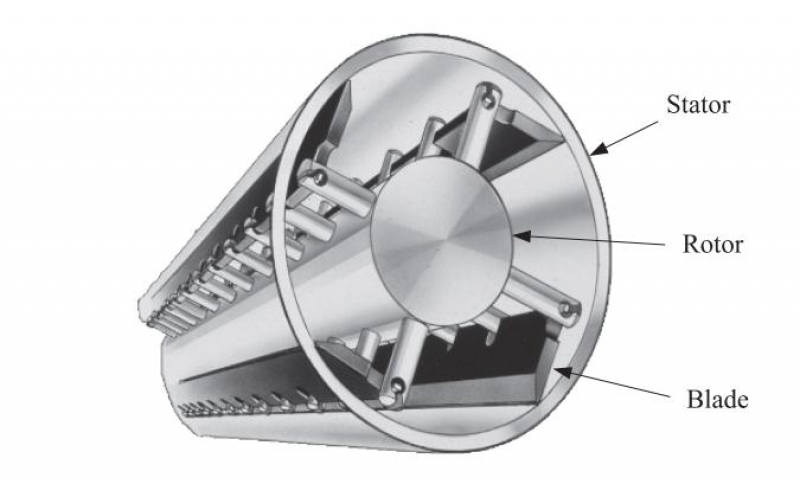
A simple mathematical model of fluid flow in a common type of scraped-surface heat exchanger in which the gaps between the blades and the device walls are narrow, so that a lubrication-theory description of the flow is valid, is presented. Specifically, steady isothermal flow of a Newtonian fluid around a periodic array of pivoted scraper blades in a channel with one stationary and one moving wall, when there is an applied pressure gradient in a direction perpendicular to the wall motion, is analysis. The flow is three-dimensional, but decomposes naturally into a two-dimensional “transverse” flow driven by the boundary motion and a “longitudinal” pressure-driven flow. First details of the structure of the transverse flow are derived, and, in particular, the equilibrium positions of the blades are calculated. It is shown that the desired contact between blades and the moving wall will be attained, provided that the blades are pivoted sufficiently close to their ends. When the desired contact is achieved, the model predicts that the forces and torques on the blades are singular, and so the model is generalized to include three additional physical effects, namely non-Newtonian power-law behavior, slip at rigid boundaries, and cavitation in regions of very low pressure, each of which is shown to resolve these singularities. Lastly the nature of the longitudinal flow is discussed.
Post time: Jun-22-2021